Star with Coefficient a in the set of Real Numbers

Mohamed Moktar Chaffar^*
*Correspondence: Mohamed Moktar Chaffar, Lycee Georges Brassens Villeneuve-le-Roi France and Temporary Professor at the University of Paris-Est Cr eteil (Paris 12) and at the Galilee Villetaneuse Institute (Paris 13), Paris, France, Tel: 0642448449, Email:

Keywords

System equation • Agebra • Linear equations • Matrix matrices

Introduction

The aim of the present paper is to introduce and study a system of five equations in five unknowns, that will be called Star-System with coefficient α in five unknowns. Let a, b, c, d, e, α be elements of a R, and let T1, T2, T3, T4, T5 be unknowns (also called variables or indeterminates). Consider a star with α coefficient (-- 1)

In addition to having the sum α in each line. The scalars α are called the star coefficient if α is a solution of equation α = T1(α) + T2(α) + T3(α) + T4(α) + T5(α) (Noted by α?), a vector (T1 , T2 , T3 , T4 , T5) is called a solution vector of this Star-System with coefficient α in five unknowns.

The present paper is organized as follows: In Section 2, we present some preliminary results and notations that will be useful in the sequel. In Section 3, we present some examples of Star-element. Section 4 is devoted to introduce and study a Star-function. In Section 5, we present one example of equivalent star-systems. Finally, in Section 6, we introduce the star-Differential operator and study some of their applications.

Some Basic Definitions and Notations

In this section, we introduce some notations and star-system with coefficient α defined.

Star with coefficient α in the set of real numbers.

Definition 1. A star with α coefficient is composed of five numbers outside a, b, c, d, e and five numbers inside T1, T2, T3, T4, T5, These last five numbers are written in the form of 5-tuple (T1, T2, T3, T4, T5) (Figure 2).

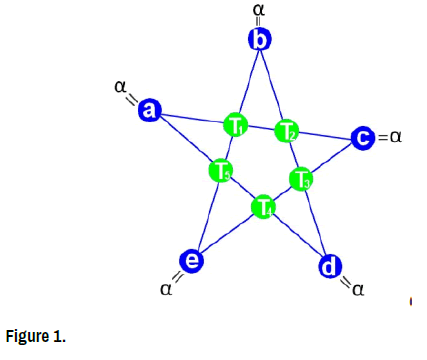
In addition to having the sum α in each line.

A star-system with coefficient α:

Definition 2. Let a, b, c, d, e and α be real numbers, and let T1, T2, T3 , T4 , T5 be unknowns (also called variables or indeterminates). Then a system of the form is called a star-system with coefficient α in five unknowns. We have also noted [a,b,c,d,e;α] = α. The scalars a, b, c, d, e are called the coefficients of the unknowns, and α is called the constant ”Chaff” of the star-system in five unknowns. A vector (T1,T2,T3,T4,T5) in R⁵ is called a star-solution vector of this star-system if and only if [a,b,c,d,e;α] = α.

The solution of a Star-system is the set of values for T1, T2, T3, T4 and T5 that satisfies five equations simultaneously.

A star-element: A star-element is a term of the five-tuple (T1, T2, T3, T4, T5) solution of a star-system [a,b,c,d,e;α] = α, where (T1, T2, T3, T4, T5)∈ R⁵.

Star-Coefficient or Constant ”Chaff”: The star-Coefficient or Constant ”Chaff” is also noted by α? and is a solution of equation 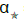= T1(α) + T0 2α) + T3(α) + T4(α) + T5(α), wher (T1 , T2 , T3 , T4 , T5) is solution of a star-system [a,b,c,d,e;α] = α.

Star-Matrix: The star-system with coefficient α can be written in matrix form

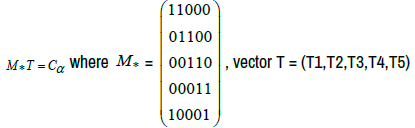
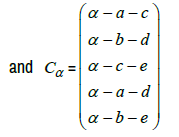
M_* or M_Staris called the star-Matrix of the star-system with coefficient α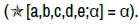

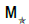a matrix is said to be of dimension 5 × 5. A value called the determinant of M , that we denote by |M_Staris| or |M|, corresponds to square matrix MF. Consequently, the determinant of Mis |M| = 1.

Set-Star: The set-star is constructed from the solution set of linear starsystem with coefficient α ( [a,b,c,d,e;α] = α). The Set-star will be noted by

Star-System equivalent: Equivalent Star-Systems are those systems having exactly same solution, i.e. Two star-systems are equivalent if solution of on starsystem is the solution of other, and vice-versa.

Parametrized Curves: A parametrized differentiable curve is simply a specific subset of R⁵ with which certain aspects of differntial calculus can be applied.

Definition 3. A parametrized differentiable curve is a differentiable map α : I →R⁵ of an open interval I = (a,b) of the real line R in to R⁵

Regular Curves: A parametrized differentiable curve α : I →R⁵, We call any point that satisfies α’(t) = 0 a singular point and we will ristrict our study to curves without singular points.

Definition 4. A parametrized differentiable curve is a differentiable α : I → R⁵ is said to be regular if α’(t) ≠ 0 for all t ∈ I

Parametric Arclength: Generalized, a parametric arclength starts with a parametric curve in R⁵. This is given by some parametric equations T1(t),T2(t),T3(t),T4(t),T5(t) , where the parameter t ranges over some given interval. The following formula computes the length of the arc between two points a, b.

Lemma 1. Consider a parametric curveT1(t),T2(t),T3(t),T4(t),T5(t), where t ∈ (a,b). The length of the arc traced by the curve as t ranges overt (a,b) is

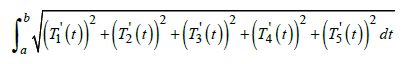
Thereafter I start with several examples with detailed solutions are presented.

Examples of Star-element

This section will deal with solving problems with star-systems of five linear equations and five variables.

Example 1. A linear star-system with coefficient α composed of five linear equations in five variables T1 , T2 , T3 , T4 and T5 has the general form [a,b,c,d,e;α] = α. In example 1: (a,b,c,d,e) = (1,2,3,4,5) When looking for the Solution of StarSystem with coefficient [1,2,3,4,5;α] = α of Linear Equations, we can easily solve this using Star-Matrix .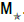

the star-systems of Linear Equations 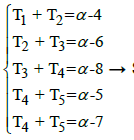So the overall solution

is the set-star: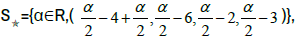

in a particular case if that is to say α=10

We obtain the following results:

• The Star-coefficient: = 10

• The star-element is (1,5,−1,3,2) (Figure 3)

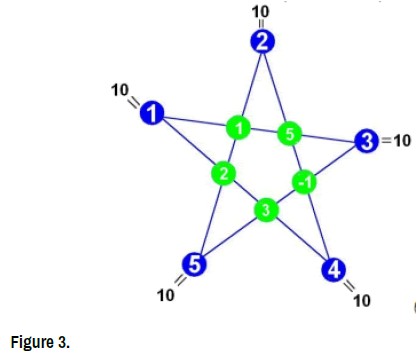
Note 1. It is important to mention that a solution is made up of five values, (T1 , T2 , T3 , T4 , T5). A solution is made up of the set of values jointly taken by the variables to satisfy the system’s equations.

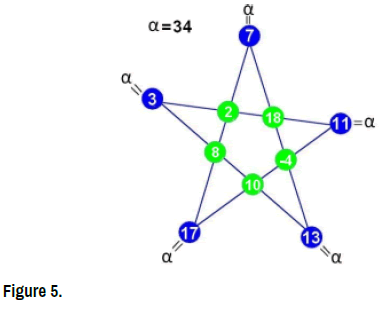
Example 2. ”Image of a five prime numbers”

Solve the following Star-system with coefficient α and five unknowns:

[3,7,11,13,17;α] = α. (Figure 4)

System [3,7,11,13,17;α] = α is therefore

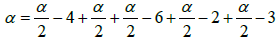So the overall solution is the star-set: S= in a particular case if Then

• The Star-coefficient: = 34

• The star-element is (2,18,−4,10,8) (Figure 5)

More generally

Theorem 1. Let α ∈R, for all (a,b,c,d,e) ∈R⁵ the star-system [a,b,c,d,e;α] = α has a unique solution and the star-system we have unique star-cofficient: = 2/3(a+b+c+d+e)

For any star-system [a,b,c,d,e;α] = α, the star-element is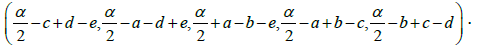

If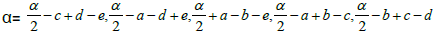

Then

• The Star-coefficient:

Examples of Star-function

In the following theorem, we give some useful result. Theorem 2: Let α ∈ R, for all t ∈ R the Star-system [t,t,t,t,t;α] = α has a unique solution, the Star-set containing only the vector (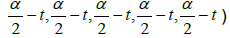 and the Star-system we have unique starcoefficient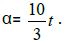

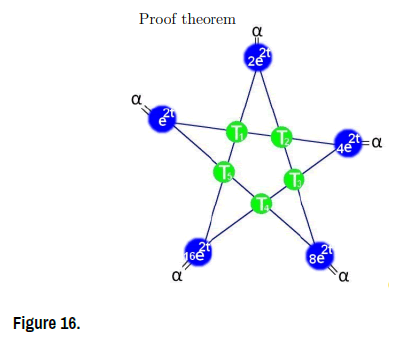
Proof theorem (Figure 6)

The star with coefficient α:

Consider the following star-system of 5 equations in 5 unknowns:

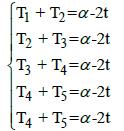
So the overall solution is the Star-set: S =

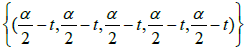
in a particular case if 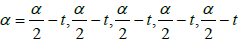Then

• The Star-coefficient: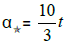

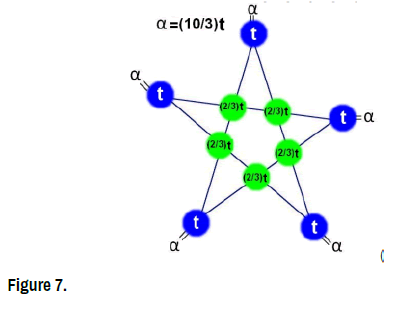
• The star-function: 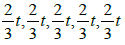(Figure 7)

Theorem 3: Let α ∈ R, for all t ∈ R the Star-system 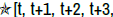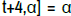has a unique solution, the Star-set containing only the vector ( 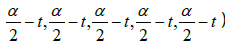and the Star-system we have unique starcoefficient :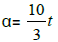

Proof theorem (Figure 8)

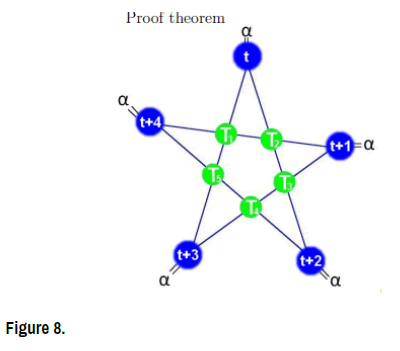
Consider the following star-system of 5 equations in 5 unknowns:

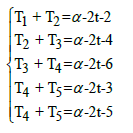
So the overall solution is the Star-set:

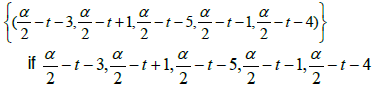
Then

• The Star-coefficient: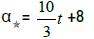

• The star-function: 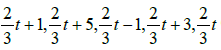(Figure 9)

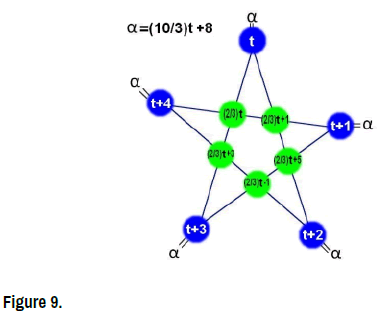
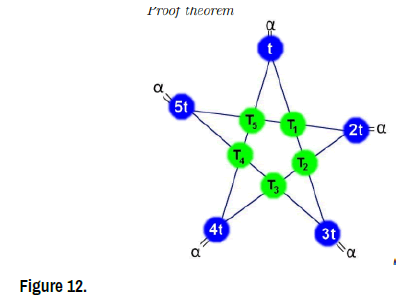
Theorem 4: Let α ∈ R, for all t ∈ R the Star-system [x,2x, 3x, 4x, 5x, α] = α has a unique solution, the Star-set containing only the vector (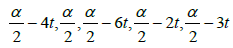) and the Star-system we have unique star-coefficient : α= 10t .

Proof theorem (Figure 10)

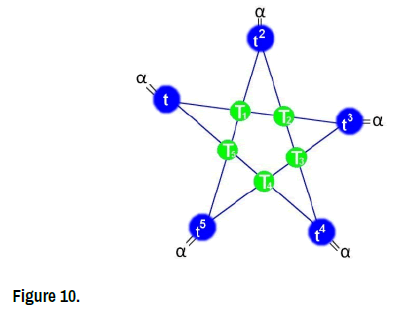
So the overall solution is the Star-set:

if Then

• The Star-coefficient: = 10t.

• The star-function: (t,5t,−t,3t,2t) (Figure 11)

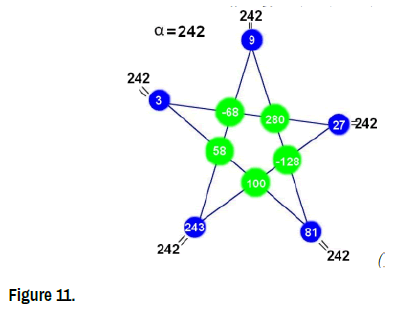
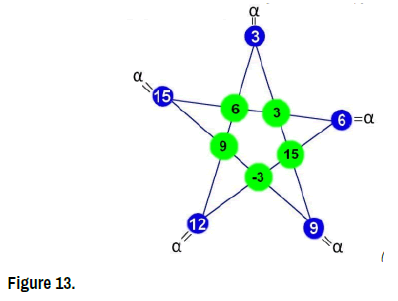
In the special case t=3

•The Constant ”Chaff”: α = 30

•The Star-set: = {3,15,−3,9,6)}. (Figure 12)

Theorem 5: Let α ∈ R, for all t ∈ R the Starsystem [t,t², t³, t⁴, t⁵,α] = α has a unique solution, 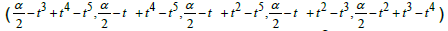and the Star-system we have unique star-coefficient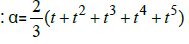

Proof theorem (Figure 13)

Star-set:

If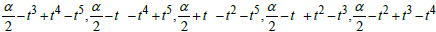

Then

• The Star-coefficient: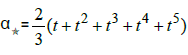

• The star-function: defined by:

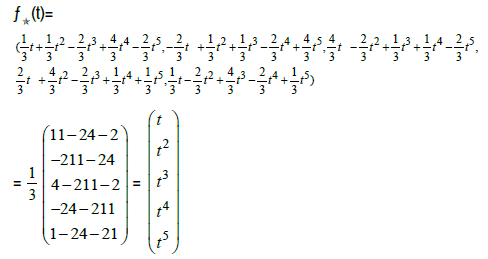
In the special case t=3

• The Star-coefficient: α = 242

• The Star-set:S= {(−68,280,−128,100,58)}.(Figure 14)

Theorem 6: Let α ∈R, for all t ∈R the Star-system 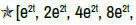has a unique solution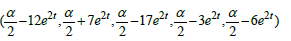 and the Star-system we have unique star-coefficient:

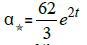
Proof theorem (Figure 15)

Consider the following star-system of 5 equations in 5 unknowns:

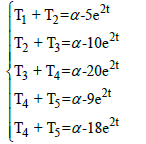
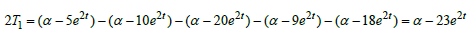
So the overall solution is the Star-set: S=

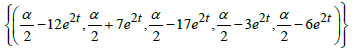
If α =T1 + T2 + T3 + T4 + T5 then the star coefficient:

Let’s find the length of of the star-function ƒ(t)= 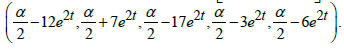We compute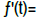 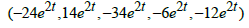and

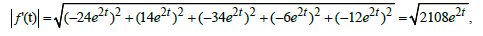
So the length is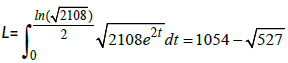

In the special case x= 0

• The star coefficient: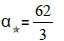

• The Star-set:

Examples of Star-systems equivalent

Theorem 7.

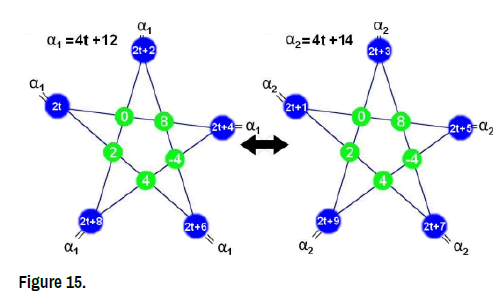
For all t ∈R if α₁ = 4t + 12 and α₂ = 4t + 12 then the two star-systems : ₁[2t,2t+2,2t+4,2t+6,2t+8;α₁] = α₁ and ₂[2t+1,2t+3,2t+5,2t+7,2t+9;α₂] = α₂ are equivalent.

Proof theorem

The star-system 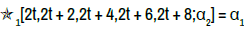can be written as:

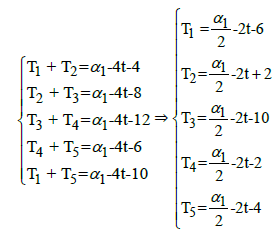
The star-system 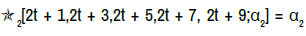can be written as:

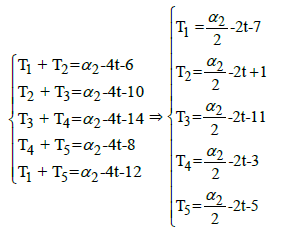
For all t ∈ R, if the tow Constants of the two star-systems α₁ = 4t +12 and 2 α = 4t+14 then the Star-set of the two star-systems: {(0,8,-4,4,2)} (Figure 16)

Examples of Star-operators

New Star-Differential operators: During our study of the construction of star-system, some new star-differential operators are required to be introduced.

Theorem 8. Let α ∈R, for all t ∈R and for all f be an n-times differentiable real function defined in interval I of R, the Star-system 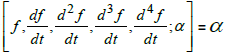has a unique solution and the Star-system we have unique star coefficient: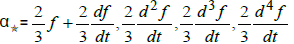

Notation: A variety of notations are used to denote the n-times derivative.

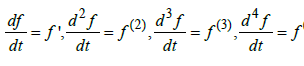(4)

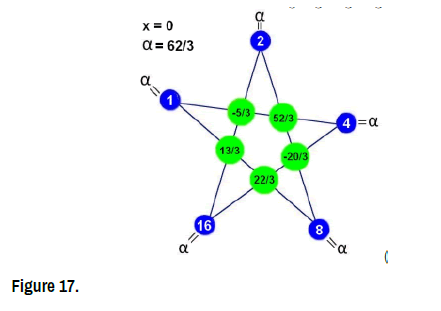
Proof theorem (Figure 17)

Consider the following star-system of 5 equations in 5 unknowns:

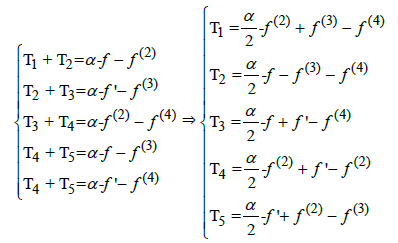
If 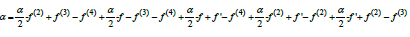then the star coefficient: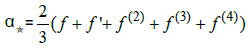

In the special case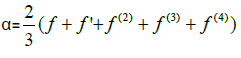

Applications

f(t)=sin(t): f is n-times differentiable at all t ∈R

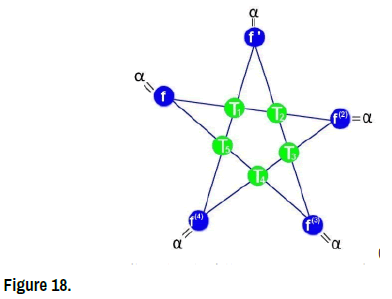
For all t ∈R the star-system [sint,cost,−sint,−cost,sint;α] = α

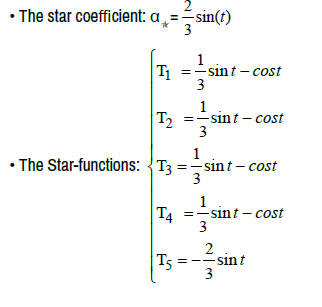
• The Star-Set: 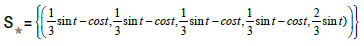(Figure 18)

f(t)=cos(t): f is n-times differentiable at all t ∈R

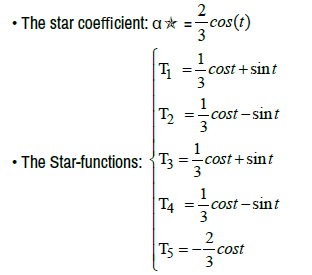
For all t ∈R the star-system [cost,−sint,−cost,sint,cost;α] = α

So the solution set is The Star-Set: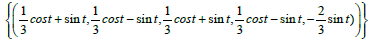

The star-function defined in R to R⁵

It’s not possible to draw a 5D graphic, but in another world, a 5D world, it would be.

which can be written

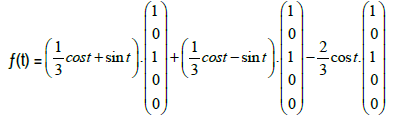
The three vectors shown span the solution star-set. it is also not too hard to prove that they are linearly independent; therefore they form a basis for the solution starset (Figure 17).

In an abstract setting we can generally say that a projection is a mapping of a set, which means that a projection is equal to its composition with itself. (Figure 18)

in our case, we define by

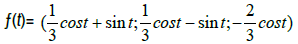the Star function in R to R³.

In our world this Star-function is represent by (Figure 19):

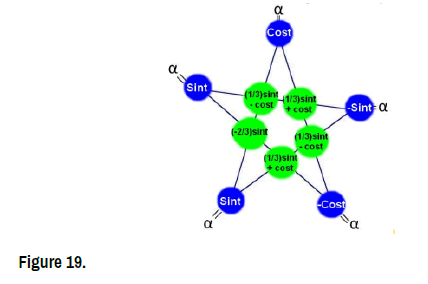
f(t)=sin(2t): f is n-times differentiable at all t ∈R For all

t ∈R the star-system [sin2t,2cos2t,−4sin2t,−8cos2t,16sin2t;α] = α

• The star coefficient: α = 2 3 (14sin(2t)−6cos2t) (Figure 20)

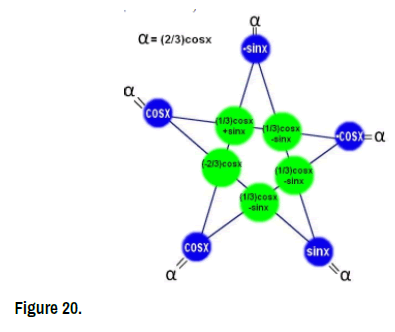
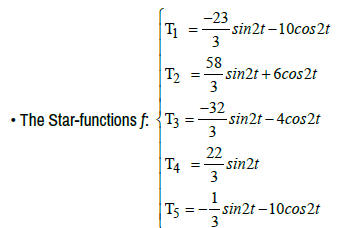
f(t)= et: f is n-times differentiable at all t ∈R (Figure 21)

For all t ∈R the star-system [e^t, e^t, e^t, e^t, e^t;α] = α

References

1. Muir, Thomas. A treatise on the theory of determinants, Revised and enlarged by William H. Metzler, New York, NY: Dover (1960) (1933).
2. Baley G. Price Some identities in the theory of determinants, American Mathematical Monthly (1947) 54:75-90.
3. Ince, EL. Ordinary differential equations, New York: Dover Publications (1956).
4. Calinger, Ronald. A Contextual History of Mathematics, Prentice Hall (1999).
5. Ahlberg, Nilson. The Theory of Splines and Their Applications, Academic Press. 51(1967).
6. Rudin, Walter. Principles of Mathematical Analysis. McGraw-Hill, Inc (1976) 137.
7. Wallis, John. Tractatus Duo. Prior, De Cycloide et de Corporibus inde Genitis. (1659) 91-96.
8. Van Heuraet, Hendrik. Epistola de transmutatione curvarum linearum in rectas Amsterdam: Louis Daniel Elzevir. (1659) 517-520.
9. Farouki, Rida T. Curves from motion, motion from curves” In Laurent (1999)
10. Schumaker, LL. Curve and Surface Design: Saint-Malo (1999). 63-90.